• 2010 June(68C) Math Question 60

    Quadratic Equations

    - Factoring

    Complex Number, Factoring cubic polynomials, Quadratic equations
    Choice J

    It is given that 2 is one root of the equation, therefore (x−2)(x-2) must be a factor of the expression 2x3−9x2+16x−122x^3-9x^2+16x-12.

    68c_math_q60a.png!

    And the new equation can be written as

    (x−2)(2x2−5x+6)=0(x-2)(2x^2-5x+6)=0

    Then we apply the Quadratic Formula to 2x2−5x+6=02x^2-5x+6=0:

    x=−b±b2−4ac2ax=\frac{-b \pm\sqrt{b^2 - 4ac}}{2a}
    x=5±52−4(2)(6)2×2x=\frac{5 \pm\sqrt{5^2 - 4(2)(6)}}{2\times2}
    x=5±−234x=\frac{5 \pm\sqrt{-23}}{4}
    x=5±23−14x=\frac{5 \pm\sqrt{23}\sqrt{-1}}{4}
    ∴x=5±23i4\therefore x=\frac{5 \pm\sqrt{23}i}{4}

    Skills you are tested for:

    Was this explanation helpful?

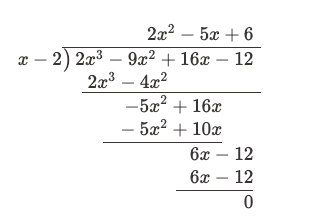
    • Comments

    To leave a comment,